Discussion/Introduction

It is imperative that students have an understanding of the logic of positive and negative exponents before they can start multiplying and dividing with them. This is a foundational lesson that dives into not only how to convert negative exponents to positive exponents but also teaches the logic behind this conversion.

Objective

Learning Objectives/Goals

• Students will be able to convert integer exponents into standard form.
• Students will have an understanding of and be able to work with negative and positive exponents.

Supplies

Supplies Required

• Base ten blocks or linking cubes (100 per group if you plan on each group building all the way to a 4 x 4 x 4)
• Multiplication table (25 x 25) (1 per student)
• Integer Exponents Intro Worksheet
• Exponents Match Up Cards (either on-level or supported version)

Methodology/Procedure

Methodology

Anticipatory Set (Building Understanding with Blocks)

• Have students work with their table groups for this activity.
• Give each table group base ten blocks (or linking cubes if you have enough)
• Have students build (as a group) squares (imagining the blocks are 1-dimensional) with the following areas:
  • 1 square unit
  • 4 square units
  • 9 square units
  • 16 square units
• Discuss how they decided to build the shapes.
• Also, discuss where these numbers appear on a multiplication table. Have students color in all numbers on the multiplication table that could result in perfect squares.
• Point out that each perfect square is made by multiplying a number by itself, or taking a number to the second power.
• Pass out the Exponents Intro Worksheet. Here’s a sample side one of this worksheet:

• Fill in the squared column for the table on the Integer Exponents Intro Worksheet.
• Next have the students construct (using the blocks already set up) cubes with the following dimensions:
  • 1 x 1 x 1
  • 2 x 2 x 2
  • 3 x 3 x 3
  • 4 x 4 x 4
• Discuss how they decided to build the shapes.
• Discuss with the students why these numbers do not appear in a straight forward pattern on a multiplication table. Guide them to the understanding that a multiplication table only has two values being multiplied together. I have included a challenge investigation in which students can use a multiplication table to find cubes.
• Point out that each perfect cube is made by multiplying a number by itself and then by itself again, or taking a number to the third power.
• Fill in the cubed column for the table on the Integer Exponents Intro Worksheet.
• As a class, have the following discussion:
  • Let’s look at the two’s row. When we have 2¹, what is our base? What is our exponent? What does this integer exponent mean? What is 2¹?
  • Would this hold true for all other integers to the first power?
    • Have students fill in the “n¹” column.
  • Can anybody see a pattern happening in the two’s row? What is the rule for the row?
    • Students should point out that each column is double (or times 2) the column to the left of it.
    • Write this rule on the worksheet for the two’s row.
  • When we have 2⁰, what is our base? What is our exponent? Let’s look at our rule for this row. What number, when we double it or multiply it by two, gives us 2?
  • Would this hold true for all other integers to the zero power?
    • Have students fill in the “n⁰” column.
  • Let’s go back to the two’s row. When we have 2^-1, what is our base? What is our exponent? Let’s look at our rule for this row. What number, when we double it or multiply it by two, gives us 1?
  • How are 2^-1 and 2¹ related?
    • Help them understand that they are reciprocals.
  • When we have 2^-2, what is our base? What is our exponent? Let’s look at our rule for this row. What number, when we double it or multiply it by two, gives us ½?
  • How are 2^-2 and 2² related?
    • Help them understand that they are reciprocals.
  • When we have 2^-3, what is our base? What is our exponent? Let’s look at our rule for this row. What number, when we double it or multiply it by two, gives us ¼?
  • How are 2^-3 and 2³ related?
    • Help them understand that they are reciprocals.
  • Guide the students through the same reasoning with the one’s row, the three’s row and the negative two’s row.
  • Have the students work with their group to complete the remaining rows. Check in with the class upon completion.
• Flip the Integers Exponents Worksheet over and discuss the alternative approach to negative exponents. Here’s a sample from side 2 of this worksheet:

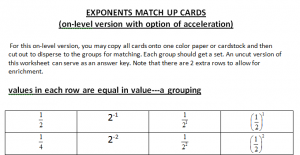
• Get students into groups of 3-5 students for the Exponents Match Up activity. There are two different versions of this activity. There is the on-level version where student groups will be given 32-40 cards (all the same color) and will be asked to make 8-10 groups comprised of 4 cards with equivalent values. The supported version can have up to 32 cards yet would be photocopied on 4 different colors of paper to help make the activity a bit easier. No grouping (in the supported version) will have more than one card of the same color. This activity does require teachers to cut the match up puzzle pieces beforehand. The filled in 4-column chart can serve as the answer key. Equivalent terms are organized by row. Here’s a sample from the on-level version:

• Go over the answers as a group to check for accuracy at the end. An alternative option, if you do not need to use the cards again for another class, you can have the students staple together the pieces or organize them on a poster paper to use this activity as a group assessment.
• Homework/Review practice can be worksheets including work with negative and positive exponents.

Download Lesson Plan

Common Core Standards

Common Core Standard Addressed:

CCSS.MATH.CONTENT.8.EE.A.1
Know and apply the properties of integer exponents to generate equivalent numerical expressions. For example, 3² × 3^-5 = 3^-3 = 1/3³ = 1/27.

Web Resources/Further Exploration

• Laws of Exponents
• Negative Exponents
• Rules of Exponents