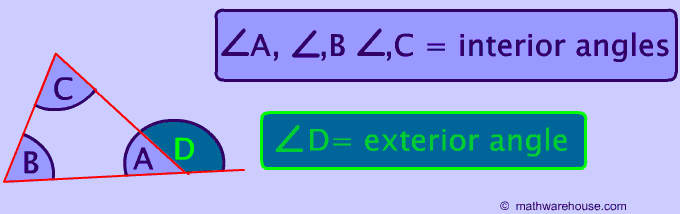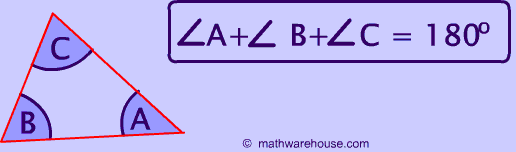Rules of a Triangle- Sides, angles, Exterior angles, Degrees and other properties

Triangle Calculator (This free online tool lets calculates all sides and angle measurements based on your input and draws a free downloadable image of your triangle!)

Triangle Worksheets

Properties of Triangles

Triangles are one of the most fundamental geometric shapes and have a variety of often studied properties including:

Rule 1: Interior Angles sum up to $$ 180^0 $$ Rule 1

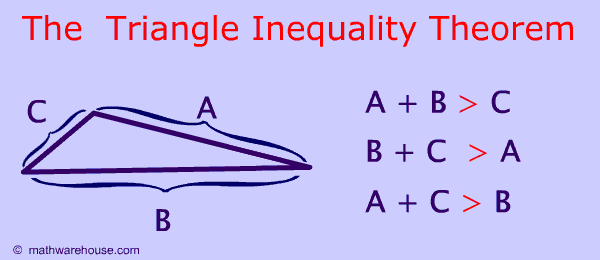
Rule 2: Sides of Triangle -- Triangle Inequality Theorem : This theorem states that the sum of the lengths of any 2 sides of a triangle must be greater than the third side. ) Rule 2

Rule 3: Relationship between measurement of the sides and angles in a triangle: The largest interior angle and side are opposite each other. The same rule applies to the smallest sized angle and side, and the middle sized angle and side. Rule 3

Rule 4 Remote Extior Angles-- This Theorem states that the measure of a an exterior angle $$ \angle A$$ equals the sum of the remote interior angles' measurements. more) Rule 4

What's the difference between interior and exterior angles of a triangle?

This question is answered by the picture below. You create an exterior angle by extending any side of the triangle.

Picture of interior and exterior angles of a triangle

Interior Angles of a Triangle Rule

This may be one the most well known mathematical rules-The sum of all 3 interior angles in a triangle is $$180^{\circ} $$. As you can see from the picture below, if you add up all of the angles in a triangle the sum must equal $$180^{\circ} $$.

To explore the truth of this rule, try Math Warehouse's interactive triangle, which allows you to drag around the different sides of a triangle and explore the relationship between the angles and sides. No matter how you position the three sides of the triangle, the total degrees of all interior angles (the three angles inside the triangle) is always 180°.

This property of a triangle's interior angles is simply a specific example of the general rule for any polygon's interior angles.

Interior Angles of Triangle Worksheet

Interior Angles Interactive Demonstration

Total	180
∠ A
∠ B
∠ C

Drag Points Of The Triangle To Start Demonstration

Practice Problems (interior angles rule)

Problem 1

What is m$$\angle$$LNM in the triangle below?

$$ \angle $$ LMN = 34°
$$ \angle $$ MLN = 29°

Use the rule for interior angles of a triangle:

m$$ \angle $$ LNM +m$$ \angle $$ LMN +m$$ \angle $$ MLN =180°
m$$ \angle $$ LNM +34° + 29° =180°
m$$ \angle $$ LNM +63° =180°
m$$ \angle $$ LNM = 180° - 63° = 117°

Problem 2

A triangle's interior angles are $$ \angle $$ HOP, $$ \angle $$ HPO and $$ \angle $$ PHO. $$ \angle $$ HOP is 64° and m$$ \angle $$ HPO is 26°.
What is m$$ \angle $$ PHO?

Use the interior angles of a triangle rule:

m$$ \angle $$ PHO = 180° - 26° -64° = 90°

Relationship --Side and Angle Angle Measurements

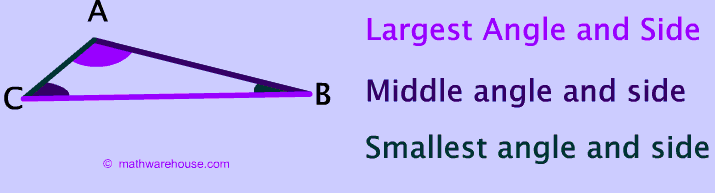
In any triangle

the largest interior angle is opposite the largest side
the smallest interior angle is opposite the smallest side
the middle-sized interior angle is opposite the middle-sized side

To explore the truth of the statements you can use Math Warehouse's interactive triangle, which allows you to drag around the different sides of a triangle and explore the relationships betwen the measures of angles and sides. No matter how you position the three sides of the triangle, you will find that the statements in the paragraph above hold true.

(All right, the isosceles and equilateral triangle are exceptions due to the fact that they don't have a single smallest side or, in the case of the equilateral triangle, even a largest side. Nonetheless, the principle stated above still holds true. !)

Worksheet on the relationship between the side lengths and angle measurements of a triangle

Triangle Calculator (This free online tool lets calculates all sides and angle measurements based on your input and draws a free downloadable image of your triangle!)

Triangle Worksheets