Quick Overview
- Limits that have the form $$0\cdot \infty$$ are indeterminate.
- L'Hôpital's Rule cannot be used on these limits directly, but we can adjust algebraically so we can use the rule.
- To adjust, rewrite one of the factors as the "reciprocal of the reciprocal." So either
$$ \begin{align*} \blue 0\cdot \red \infty & = \blue{\frac 1 {1/0}} \cdot \red \infty = \frac{\red\infty}{\blue{1/0}} = \frac{\red\infty}{\blue \infty}\\[6pt] &\quad \mbox{or}\\ \blue 0\cdot \red \infty & = \blue 0 \cdot \red{\frac 1 {1/\infty}} = \frac{\blue 0}{\red{1/\infty}} = \frac{\blue 0}{\red 0} \end{align*} $$
Introduction---Why Is The $$0\cdot \infty$$ Form Indeterminate?
Suppose we need to evaluate $$ \displaystyle \lim_{x\to a} \blue{f(x)}\red{g(x)} $$, but when we try, we find that $$ \blue{f(x) \to 0} $$ and $$ \red{g(x) \to \infty} $$, so $$ \displaystyle \lim_{x\to a} \blue{f(x)}\red{g(x)} = \blue 0 \cdot \red \infty $$. But what does that mean?
Since we're evaluating a limit, this tells us

If $$ \blue{f(x)} $$ is getting smaller, and $$ \red{g(x)} $$ is getting larger, what happens to their product?
The answer is, it depends on how quickly $$ \blue f $$ is getting smaller compared to how quickly $$ \red g $$ is getting larger. This is what makes the limit indeterminate.
How to Adjust the Function for L'Hôpital's Rule
In order to use L'Hôpital's Rule we use the following two ideas:
- If $$ \lim\limits_{x\to a} \blue{f(x)} = \blue 0 $$ then $$ \lim\limits_{x\to a} \frac 1 {\blue{f(x)}} = \frac 1 {\blue 0} = \infty $$. Similarly, if $$ \lim\limits_{x\to a} \red{g(x)} = \red \infty $$ then $$ \lim\limits_{x \to a} \frac 1 {\red{g(x)}} = \red 0 $$.
- $$ \blue{f(x)} = \frac 1 {1/\blue{f(x)}} $$, and $$ \red{g(x)} = \frac 1 {1/\red{g(x)}} $$.
Combining these to ideas leads us to the following technique. If $$ \lim\limits_{x\to a} \blue{f(x)}\red{g(x)} = \blue 0\cdot \red \infty $$ then either
$$ \begin{align*} \lim_{x\to a} \blue{f(x)}\,g(x) & = \lim_{x\to a} \blue{\frac 1 {1/f(x)}} \cdot g(x) = \lim_{x\to a} \frac{g(x)}{\blue{1/f(x)}} = \frac \infty \infty,\\[6pt] \text{or } \lim_{x\to a} f(x)\,\red{g(x)} & = \lim_{x\to a} f(x) \cdot \red{\frac 1 {1/g(x)}} = \lim_{x\to a} \frac{f(x)}{\red{1/g(x)}} = \frac 0 0. \end{align*} $$
Either way, we transform the limit into one that L'Hôpital's Rule can handle!
Examples
Example 1
Evaluate $$ \lim\limits_{x\to\infty} xe^{-x} $$.
Step 1Evaluate the function in its current form.
$$ \displaystyle\lim_{x\to\infty} \blue x \red{e^{-x}} = \blue \infty\cdot \red 0 $$
Step 2Rewrite the function in $$ \frac \infty \infty $$ form.
$$ \begin{align*} \lim_{x\to\infty} x \red{e^{-x}} & = \lim_{x\to\infty} x \cdot \red{\frac 1 {1/e^{-x}}}\\[6pt] & = \lim_{x\to\infty} x \cdot \frac 1 {\red{e^x}} && \text{Simplifying at each stage is a good idea!}\\[6pt] & = \lim_{x\to\infty} \frac x {\red{e^x}}\\[6pt] & = \frac \infty {e^\infty} && \href{../limits/what-is-a-limit.php}{\text{Evaluating the limit}}\\[6pt] & = \frac \infty \infty \end{align*} $$
Step 3Now use L'Hôpital's Rule and re-evaluate the limit.
$$ \begin{align*} \lim_{x\to\infty} \frac x {e^x} & = \lim_{x\to\infty} \frac{\blue{\frac d {dx}(x)}}{\red{\frac d {dx}(e^x)}}\\[6pt] & = \lim_{x\to\infty} \frac{\blue 1}{\red{e^x}}\\[6pt] & = \frac 1 {e^\infty} && \href{../limits/what-is-a-limit.php}{\text{Evaluating the limit}}\\[6pt] & = \frac 1 \infty \end{align*} $$
This limit indicates the denominator is growing arbitrarily large, while the numerator is constant. A fraction with this behavior approaches 0.
Answer$$\lim\limits_{x\to\infty} xe^{-x}= 0$$
For reference, here is the graph of the function.

HOW DID WE Choose?
In Step 2 of Example 1, we had to make a choice when we rewrote the function. We could have chosen either
$$ \begin{align*} \lim_{x\to\infty} x\,\red{e^{-x}} & = \lim_{x\to\infty} \frac x {\red{1/e^{-x}}} = \frac \infty \infty\\[6pt] &\quad \mbox{or}\\ \lim_{x\to\infty} \blue x e^{-x} & = \lim_{x\to\infty} \frac{e^{-x}}{\blue{1/x}} = \frac 0 0 \end{align*} $$
The choice we made came from a little forethought. Consider what would have happened if we had chosen the second version and applied L'Hôpital's rule:
$$ \begin{align*} \lim_{x\to\infty} \blue x\,e^{-x} & = \lim_{x\to\infty} \frac{e^{-x}}{\blue{1/x}} &\text{Rewriting}\\[6pt] & = \lim_{x\to\infty} \frac{-e^{-x}}{\blue{-1/x^2}} & \href{what-is-Lhopitals-rule.php}{\text{L'Hôpital's rule}} \\ \\ & \lim_{x\to\infty} \blue{x^2}\,e^{-x} &\text{Simplifying} \end{align*} $$
We've gone "backwards" in some sense. We've gone from $$ \blue x\,e^{-x} $$ to $$ \blue{x^2}\,e^{-x} $$. Further applications would simply increase the power on $$ x $$. By thinking ahead, we could see this happening with each successive application of L'Hôpital's rule:
$$ \blue x\,e^{-x} \longrightarrow \blue{x^2}e^{-x} \longrightarrow \frac 1 2 \blue{x^3}e^{-x} \longrightarrow \frac 1 6 \blue{x^4}e^{-x} \longrightarrow\ldots $$
This is an important point! Sometimes it matters a great deal which of the two factors you put into the denominator! If you're not sure, just pick one version and try it. If it doesn't work out, start over with the other version and try again.
Example 2
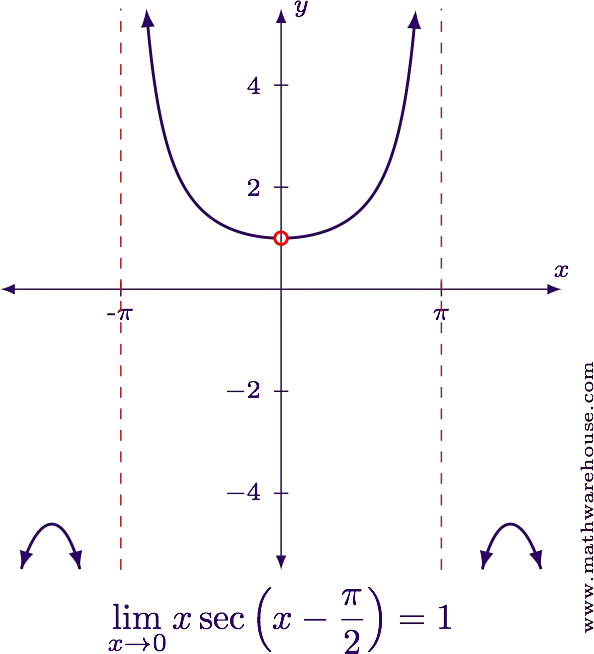
Evaluate $$ \displaystyle \lim\limits_{x\to 0} x\sec\left(x-\frac \pi 2\right) $$.
Step 1Evaluate (carefully) the limit in its current form.
When we look at the graph of $$ y = \sec\left(x - \frac \pi 2\right) $$ near $$ x = 0 $$ we see that has different behavior depending on whether we approach $$ x = 0 $$ from the left or from the right. Consequently, let's examine our limit using one-sided limits initially.

Limit from the Left
$$ \displaystyle\lim_{x\to 0^-} \blue x\,\red{\sec\left(x-\frac \pi 2\right)} = \blue{-0}\cdot\red{(-\infty)} = 0 \cdot \infty $$
where "$$ \blue{-0} $$" indicates that $$ \blue x $$ is negative as it approaches zero.
Limit from the Right
$$ \displaystyle\lim_{x\to 0^+} \blue x\,\red{\sec\left(x-\frac \pi 2\right)} = \blue{+0}\cdot\red{+\infty} = 0 \cdot \infty $$
again, where "$$ \blue{+0} $$" indicates $$ \blue x $$ is positive as it approaches zero.
Both one-sided limits result in the $$ 0\cdot \infty $$ form, so we proceed to adjust the form of the function so we can use L'Hôpital's rule.
Step 2Determine which form ($$ 0/0 $$ or $$ \infty/\infty $$) to use.
- Choice 1: Leave the secant alone, and move the $$x$$.
Thinking ahead: We'll be taking derivatives of the secant (and maybe more than one). This will end up with powers of secants and tangents and product rules... (shudder) Maybe we can avoid all that work.
- Choice 2: Move the secant, leave the $$x$$ alone.
Thinking ahead: This means our denominator will be 1/secant. But that's just a cosine, and the derivatives of cosines just bounce back and forth between sines and cosines. Easy!
Based on our thinking ahead, let's use Choice 2 and move the secant.
Step 3Rewrite the limit and re-evaluate it.
$$ \begin{align*} \lim_{x\to 0} x\,\red{\sec\left(x-\frac \pi 2\right)} & = \lim_{x\to 0} \frac x {\red{1 / \sec\left(x-\frac \pi 2\right)}}\\[6pt] & = \lim_{x\to 0} \frac x {\cos\left(x-\frac \pi 2\right)} && \text{Simplify}\\[6pt] & = \frac 0 {\cos\left(0-\frac \pi 2\right)} && \href{../limits/what-is-a-limit.php}{\text{Evaluating the limit}}\\[6pt] & = \frac 0 {\cos\left(-\frac \pi 2\right)}\\[6pt] & = \frac 0 0 \end{align*} $$
Step 4Use L'Hôpital's rule and re-evaluate the limit.
$$ \begin{align*} \lim_{x\to 0} \frac x {\cos\left(x-\frac \pi 2\right)} & = \lim_{x\to 0} \frac{\blue{\frac d {dx} (x)}}{\red{\frac d {dx} \left(\cos\left(x-\frac \pi 2\right)\right)}}\\[6pt] & = \lim_{x\to 0} \frac{\blue 1}{\red{-\sin\left(x-\frac \pi 2\right)}}\\[6pt] & = -\frac 1 {\sin\left(0-\frac \pi 2\right)}\\[6pt] & = -\frac 1 {-1}\\[6pt] & = 1 \end{align*} $$
Answer$$\displaystyle \lim\limits_{x\to 0} x\sec\left(x-\frac \pi 2\right) = 1$$
For reference, here is the graph of the function and the limit value.