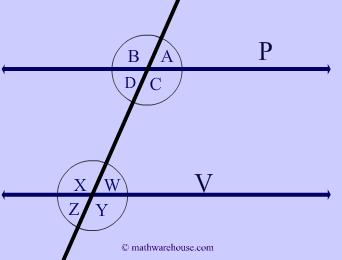
What is a transversal?
Answer: A transversal is a line, like the red one below, that intersects two other lines.
Typically, the intercepted lines like line a and line b shown above above are parallel, but they do not have to be.
What is so special about a transversal?
Answer: When a transversal cuts (or intersects) parallel lines several pairs of congruent and supplementary angles are formed.
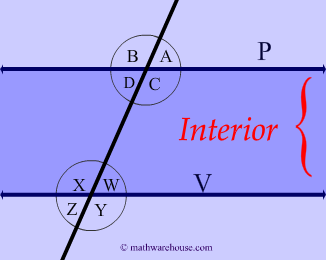
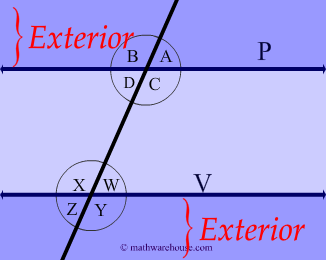
Interior and Exterior Regions
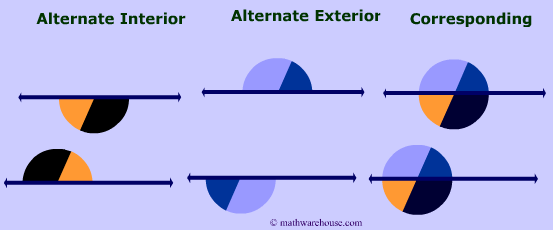
The Congruent Angle Pairs
There are 3 types of angles that are congruent: Alternate Interior, Alternate Exterior and Corresponding Angles.
Can you make a Z?
A way to help identify the alternate interior angles.
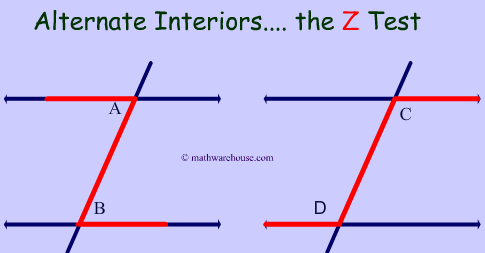
Some people find it helpful to use the 'Z test' for alternate interior angles.
If you can draw a Z or a 'Backwards Z' , then the alternate interior angles are the ones that are in the corners of the Z
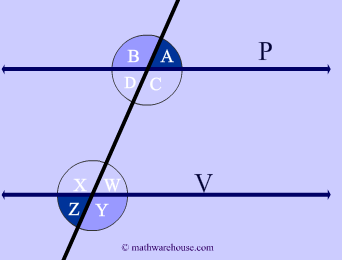
Problem 1
Line $$\overline P $$ is parallel to line $$ \overline V $$
Directions: Identify the alternate interior angles.
$$ \angle$$D and $$ \angle$$W
$$ \angle$$X and $$ \angle$$C
Problem 2
Line $$\overline P $$ is parallel to line $$ \overline V $$
Directions: Identify the alternate exterior angles.
$$ \angle$$A and $$ \angle$$Z
$$ \angle$$Y and $$ \angle$$B
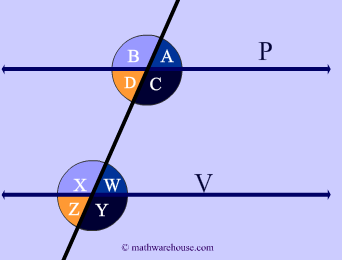
Problem 3
Line $$\overline P $$ is parallel to line $$ \overline V $$
Directions: Identify the corresponding angles.
$$ \angle$$A and $$ \angle$$W
$$ \angle$$D and $$ \angle$$Z
$$ \angle$$X and $$ \angle$$B
$$ \angle$$C and $$ \angle$$Y
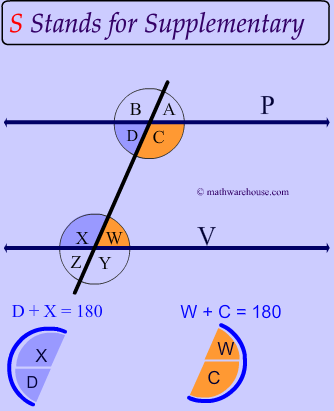
The Supplementary Angle Pairs
There are 2 types of supplementary angles that are formed: same side interior and same side exterior.
Interactive Parallel Line and Angles
Explore the rules for the different types of congruent and supplementary angles here by dragging the points and selecting which angle pair you'd like to explore.
You can create a customized shareable link (at bottom) that will remember the exact state of the app--which angles are selected and where the points are, so that you can share your it with others.
| ∠A | 90 | ∠W | 90 |
|---|---|---|---|
| ∠B | 90 | ∠X | 90 |
| ∠C | 90 | ∠Y | 90 |
| ∠D | 90 | ∠Z | 90 |
